Skewed Stem And Leaf Plot
Stem and leaf plots
Archived Content
Data identified as archived is provided for reference, enquiry or recordkeeping purposes. It is not subject to the Government of Canada Web Standards and has not been altered or updated since it was archived. Delight contact us to request a format other than those available.
- Elements of a good stem and foliage plot
- Tips on how to describe a stalk and leaf plot
- Example 1 – Making a stalk and leaf plot
- The chief advantage of a stem and leaf plot
- Example ii – Making a stem and leaf plot
- Example 3 – Making an ordered stem and leafage plot
- Splitting the stems
- Instance 4 – Splitting the stems
- Instance 5 – Splitting stems using decimal values
- Outliers
- Features of distributions
- Using stem and leaf plots as graphs
- Example six – Using stem and leafage plots as graph
A stem and leafage plot, or stem plot, is a technique used to classify either discrete or continuous variables. A stem and leaf plot is used to organize data as they are nerveless.
A stem and foliage plot looks something similar a bar graph. Each number in the data is broken downwards into a stem and a leaf, thus the proper name. The stem of the number includes all but the last digit. The leaf of the number will always exist a single digit.
Elements of a good stem and leafage plot
A proficient stalk and leafage plot
- shows the first digits of the number (thousands, hundreds or tens) equally the stem and shows the last digit (ones) every bit the leafage.
- usually uses whole numbers. Anything that has a decimal point is rounded to the nearest whole number. For example, test results, speeds, heights, weights, etc.
- looks like a bar graph when information technology is turned on its side.
- shows how the data are spread—that is, highest number, lowest number, most common number and outliers (a number that lies exterior the master grouping of numbers).
![]()
Top of Page
Tips on how to draw a stem and leaf plot
In one case you have decided that a stem and foliage plot is the best way to show your data, draw information technology as follows:
- On the left mitt side of the page, write downward the thousands, hundreds or tens (all digits only the concluding one). These volition be your stems.
- Draw a line to the correct of these stems.
- On the other side of the line, write downwards the ones (the concluding digit of a number). These volition be your leaves.
For example, if the observed value is 25, then the stem is ii and the leaf is the five. If the observed value is 369, then the stalk is 36 and the foliage is ix. Where observations are accurate to one or more decimal places, such as 23.7, the stem is 23 and the foliage is 7. If the range of values is as well great, the number 23.7 can be rounded upwards to 24 to limit the number of stems.
In stem and leaf plots, tally marks are not required because the bodily data are used.
Not quite getting it? Try some exercises.
Example 1 – Making a stalk and leafage plot
Each morning, a teacher quizzed his class with 20 geography questions. The class marked them together and everyone kept a record of their personal scores. As the year passed, each educatee tried to improve his or her quiz marks. Every day, Elliot recorded his quiz marks on a stem and leafage plot. This is what his marks looked like plotted out:
| Stalk | Leafage |
|---|---|
| 0 | 3 6 5 |
| 1 | 0 one four 3 5 vi v half dozen 8 9 7 9 |
| two | 0 0 0 0 |
Analyse Elliot's stem and leaf plot. What is his virtually common score on the geography quizzes? What is his highest score? His everyman score? Rotate the stem and leaf plot onto its side and so that it looks similar a bar graph. Are most of Elliot'south scores in the 10s, 20s or under x? It is difficult to know from the plot whether Elliot has improved or not because we do not know the order of those scores.
Try making your own stem and leaf plot. Apply the marks from something like all of your exam results last twelvemonth or the points your sports team accumulated this season.
![]()
Top of Folio
The main advantage of a stem and foliage plot
The primary advantage of a stem and leaf plot is that the information are grouped and all the original data are shown, too. In Case 3 on battery life in the Frequency distribution tables section, the table shows that two observations occurred in the interval from 360 to 369 minutes. All the same, the table does not tell you what those bodily observations are. A stem and foliage plot would show that data. Without a stem and leafage plot, the 2 values (363 and 369) tin only exist found by searching through all the original information—a ho-hum chore when you take lots of information!
When looking at a data set up, each ascertainment may exist considered as consisting of two parts—a stem and a leaf. To make a stem and foliage plot, each observed value must first exist separated into its two parts:
- The stem is the get-go digit or digits;
- The leaf is the last digit of a value;
- Each stem can consist of any number of digits; but
- Each leaf tin can have only a single digit.
![]()
Top of Page
Example 2 – Making a stem and leaf plot
A teacher asked 10 of her students how many books they had read in the final 12 months. Their answers were every bit follows:
12, 23, nineteen, 6, x, 7, 15, 25, 21, 12
Prepare a stem and leafage plot for these data.
Tip: The number 6 can be written as 06, which ways that it has a stem of 0 and a foliage of vi.
The stem and leaf plot should await like this:
| Stem | Leaf |
|---|---|
| 0 | half dozen 7 |
| i | 2 9 0 5 2 |
| two | 3 5 1 |
In Table two:
- stem 0 represents the class interval 0 to nine;
- stem 1 represents the form interval 10 to nineteen; and
- stem 2 represents the grade interval twenty to 29.
Usually, a stem and leaf plot is ordered, which merely means that the leaves are arranged in ascending order from left to right. Also, there is no need to carve up the leaves (digits) with punctuation marks (commas or periods) since each foliage is always a single digit.
Using the information from Tabular array 2, we made the ordered stem and leaf plot shown below:
| Stem | Leaf |
|---|---|
| 0 | 6 7 |
| 1 | 0 2 two five 9 |
| 2 | 1 3 v |
![]()
Acme of Page
Example 3 – Making an ordered stem and leafage plot
Fifteen people were asked how oft they drove to work over x working days. The number of times each person drove was as follows:
5, 7, ix, 9, 3, v, one, 0, 0, four, 3, 7, ii, 9, 8
Brand an ordered stalk and leafage plot for this table.
It should be fatigued equally follows:
| Stem | Foliage |
|---|---|
| 0 | 0 0 1 2 3 3 iv five v 7 7 viii 9 nine nine |
Splitting the stems
The organization of this stem and leaf plot does not give much information about the data. With only one stalk, the leaves are overcrowded. If the leaves become too crowded, so it might be useful to split each stem into two or more components. Thus, an interval 0–9 tin be separate into ii intervals of 0–four and 5–9. Similarly, a 0–ix stem could be split into 5 intervals: 0–1, 2–iii, four–5, vi–7 and 8–nine.
The stem and leafage plot should then wait like this:
| Stem | Leaf |
|---|---|
| 0(0) | 0 0 1 2 three iii 4 |
| 0(5) | v 5 7 7 8 9 nine 9 |
Note: The stem 0(0) means all the data within the interval 0–4. The stem 0(v) means all the data within the interval 5–9.
![]()
Top of Page
Example 4 – Splitting the stems
Britney is a swimmer grooming for a competition. The number of l-metre laps she swam each twenty-four hours for 30 days are every bit follows:
22, 21, 24, 19, 27, 28, 24, 25, 29, 28, 26, 31, 28, 27, 22, 39, twenty, 10, 26, 24, 27, 28, 26, 28, xviii, 32, 29, 25, 31, 27
- Ready an ordered stalk and leaf plot. Make a brief comment on what it shows.
- Redraw the stem and leaf plot by splitting the stems into 5-unit of measurement intervals. Brand a brief annotate on what the new plot shows.
Answers
- The observations range in value from 10 to 39, so the stem and leaf plot should take stems of 1, 2 and 3. The ordered stalk and leaf plot is shown beneath:
The stem and leaf plot shows that Britney usually swims between 20 and 29 laps in grooming each day.Tabular array six. Laps swum by Britney in thirty days Stem Leaf 1 0 8 9 2 0 one 2 two 4 4 4 five 5 6 6 6 seven 7 7 7 8 eight 8 viii 8 9 9 3 one 1 2 9 - Splitting the stems into five-unit intervals gives the post-obit stem and foliage plot:
Table 7. Laps swum past Britney in 30 days Stalk Leaf i(0) 0 1(5) eight nine 2(0) 0 one 2 ii iv four 4 2(v) 5 5 6 6 6 7 7 seven 7 8 8 eight eight 8 9 9 three(0) one 1 2 iii(5) nine Annotation: The stalk one(0) ways all data betwixt ten and fourteen, 1(5) means all information between xv and 19, and and so on.
The revised stem and leaf plot shows that Britney usually swims between 25 and 29 laps in grooming each day. The values 1(0) 0 = x and 3(v) nine = 39 could be considered outliers—a concept that will exist described in the next section.
![]()
Height of Page
Example five – Splitting stems using decimal values
The weights (to the nearest tenth of a kilogram) of 30 students were measured and recorded equally follows:
59.2, 61.five, 62.iii, 61.4, threescore.ix, 59.8, threescore.5, 59.0, 61.i, sixty.7, 61.6, 56.3, 61.ix, 65.7, 60.iv, 58.9, 59.0, 61.2, 62.1, 61.4, 58.4, 60.8, 60.2, 62.seven, sixty.0, 59.3, 61.9, 61.7, 58.four, 62.2
Fix an ordered stem and leafage plot for the information. Briefly annotate on what the analysis shows.
Answer
In this case, the stems will be the whole number values and the leaves will be the decimal values. The information range from 56.iii to 65.7, so the stems should starting time at 56 and finish at 65.
| Stem | Leaf |
|---|---|
| 56 | iii |
| 57 | |
| 58 | 4 4 9 |
| 59 | 0 0 ii three 8 |
| 60 | 0 2 4 5 7 eight 9 |
| 61 | 1 ii 4 4 5 6 seven 9 9 |
| 62 | i 2 3 7 |
| 63 | |
| 64 | |
| 65 | seven |
In this example, it was not necessary to split stems because the leaves are not crowded on besides few stems; nor was it necessary to circular the values, since the range of values is not large. This stalk and leaf plot reveals that the group with the highest number of observations recorded is the 61.0 to 61.ix group.
![]()
Tiptop of Page
Outliers
An outlier is an farthermost value of the information. It is an observation value that is significantly different from the rest of the data. There may be more than one outlier in a ready of data.
Sometimes, outliers are significant pieces of information and should not exist ignored. Other times, they occur because of an error or misinformation and should exist ignored.
In the previous example, 56.3 and 65.7 could be considered outliers, since these 2 values are quite different from the other values.
By ignoring these ii outliers, the previous instance's stem and leaf plot could be redrawn as below:
| Stem | Foliage |
|---|---|
| 58 | 4 four nine |
| 59 | 0 0 2 3 viii |
| 60 | 0 2 4 5 vii 8 9 |
| 61 | 1 2 4 four 5 half-dozen vii nine 9 |
| 62 | ane 2 3 7 |
When using a stem and foliage plot, spotting an outlier is often a thing of judgment. This is because, except when using box plots (explained in the section on box and whisker plots), there is no strict rule on how far removed a value must be from the residue of a information set to qualify as an outlier.
![]()
Top of Page
Features of distributions
When you assess the overall pattern of whatsoever distribution (which is the design formed past all values of a particular variable), look for these features:
- number of peaks
- general shape (skewed or symmetric)
- center
- spread
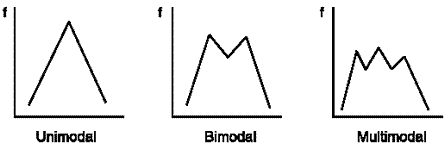
Number of peaks
Line graphs are useful because they readily reveal some characteristic of the data. (See the department on line graphs for details on this type of graph.)
The first characteristic that can be readily seen from a line graph is the number of high points or peaks the distribution has.
While most distributions that occur in statistical data accept but one main summit (unimodal), other distributions may have two peaks (bimodal) or more than two peaks (multimodal).
Examples of unimodal, bimodal and multimodal line graphs are shown below:
General shape
The second main feature of a distribution is the extent to which it is symmetric.
A perfectly symmetric curve is i in which both sides of the distribution would exactly match the other if the effigy were folded over its central indicate. An instance is shown below:
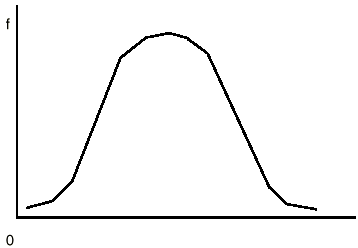
A symmetric, unimodal, bell-shaped distribution—a relatively common occurrence—is called a normal distribution.
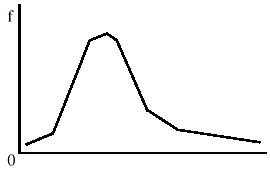
If the distribution is lop-sided, information technology is said to exist skewed.
A distribution is said to be skewed to the right, or positively skewed, when most of the data are concentrated on the left of the distribution. Distributions with positive skews are more mutual than distributions with negative skews.
Income provides one example of a positively skewed distribution. Nearly people make under $40,000 a year, but some make quite a flake more, with a smaller number making many millions of dollars a year. Therefore, the positive (correct) tail on the line graph for income extends out quite a long fashion, whereas the negative (left) skew tail stops at cipher. The right tail clearly extends farther from the distribution's centre than the left tail, equally shown beneath:
A distribution is said to be skewed to the left, or negatively skewed, if most of the information are concentrated on the right of the distribution. The left tail clearly extends farther from the distribution'southward centre than the right tail, as shown beneath:

Centre and spread
Locating the heart (median) of a distribution can be done by counting half the observations up from the smallest. Obviously, this method is impracticable for very big sets of data. A stem and leaf plot makes this like shooting fish in a barrel, notwithstanding, because the data are arranged in ascending order. The mean is another measure of cardinal tendency. (See the chapter on central tendency for more particular.)
The amount of distribution spread and any large deviations from the general pattern (outliers) tin exist apace spotted on a graph.
Using stem and leaf plots equally graphs
A stem and leaf plot is a simple kind of graph that is made out of the numbers themselves. Information technology is a means of displaying the master features of a distribution. If a stem and leaf plot is turned on its side, it will resemble a bar graph or histogram and provide similar visual information.
![]()
Top of Page
Example 6 – Using stalk and leaf plots every bit graph
The results of 41 students' math tests (with a best possible score of 70) are recorded below:
31, 49, 19, 62, 50, 24, 45, 23, 51, 32, 48, 55, 60, forty, 35, 54, 26, 57, 37, 43, 65, 50, 55, 18, 53, 41, 50, 34, 67, 56, 44, four, 54, 57, 39, 52, 45, 35, 51, 63, 42
- Is the variable detached or continuous? Explain.
- Gear up an ordered stalk and foliage plot for the data and briefly describe what it shows.
- Are there any outliers? If so, which scores?
- Look at the stem and foliage plot from the side. Describe the distribution's main features such as:
- number of peaks
- symmetry
- value at the centre of the distribution
Answers
- A exam score is a detached variable. For example, it is not possible to have a test score of 35.74542341....
- The lowest value is four and the highest is 67. Therefore, the stem and leaf plot that covers this range of values looks like this:
Table 10. Math scores of 41 students Stem Leaf 0 four 1 8 ix ii three four half-dozen 3 1 2 four 5 five 7 9 4 0 1 2 3 four 5 five 8 9 5 0 0 0 1 1 2 3 four 4 5 five 6 7 7 6 0 2 3 five 7 Notation: The annotation 2|4 represents stem two and leaf 4.
The stem and leaf plot reveals that most students scored in the interval between 50 and 59. The big number of students who obtained loftier results could mean that the test was too easy, that most students knew the fabric well, or a combination of both.
- The consequence of 4 could be an outlier, since there is a large gap between this and the next result, 18.
- If the stalk and leaf plot is turned on its side, it will look like the post-obit:
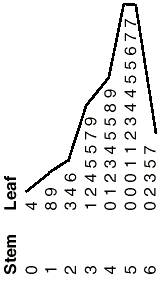
The distribution has a unmarried peak within the l–59 interval.
Although in that location are only 41 observations, the distribution shows that most data are clustered at the right. The left tail extends farther from the information centre than the right tail. Therefore, the distribution is skewed to the left or negatively skewed.
Since there are 41 observations, the distribution centre (the median value) volition occur at the 21st ascertainment. Counting 21 observations upward from the smallest, the centre is 48. (Note that the same value would have been obtained if 21 observations were counted down from the highest ascertainment.)
Skewed Stem And Leaf Plot,
Source: https://www150.statcan.gc.ca/n1/edu/power-pouvoir/ch8/5214816-eng.htm
Posted by: scottwhounces1938.blogspot.com


0 Response to "Skewed Stem And Leaf Plot"
Post a Comment